Bouncing Barney
By: Carly Cantrell
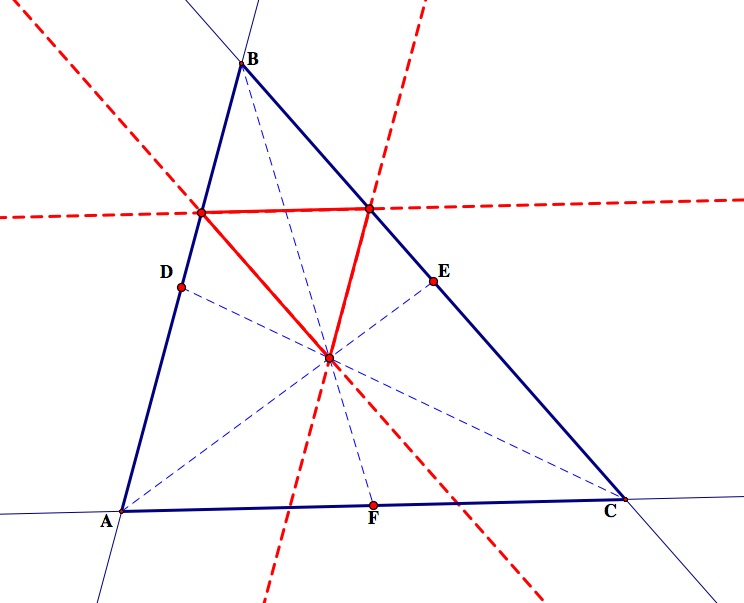
Barney is in the triangular room shown here. He walks from a
point on BC parallel to AC. When he reaches AB, he turns and walks parallel to
BC. When he reaches AC, he turns and walks parallel to AB.
Feel free to use the following GSP file to explore:
1. Prove that Barney will eventually return to his starting
position:
Note: Base of the triangle is segment 1.
Left side of the triangle is segment 2.
Right side of the triangle is segment 3.
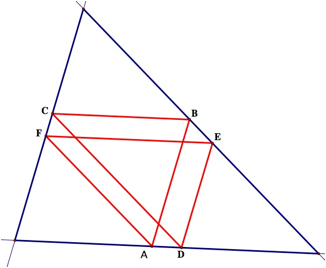
Barney will begin at point A, on segment 1. Barney bounces
to point B; while he bounced he was parallel to segment 2. Next, he bounces to
point C; while he bounced this time he was parallel to segment 1. From point C
he bounced to point D. During this bounce he was parallel to segment 3. After
D, Barney bounces to point E and he traveled parallel to segment 2. Then Barney
bounced to F and he went parallel to segment 1. Lastly, he went from F back to
A, while traveling parallel toÉcan you guess which segment? Yep, segment 3.
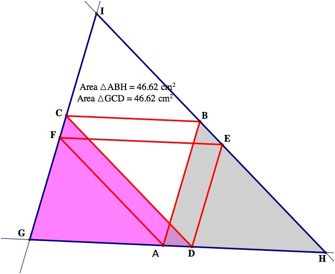
After this bouncing around, there were several
parallelograms formed. LetŐs look at the two below:
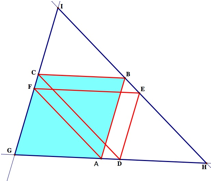
We can use the properties of parallelograms to prove that
there are congruent angles, to ultimately prove there are congruent triangles.
See, angle G is the same as angle BAH and the same as angle C. Thus by the
triangle congruence postulate, AAS, triangle GCD and ABH are congruent. I have
shown with GSP that in fact the areas are equivalent.
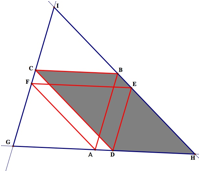
If you continue along BarneyŐs path the same processes
occur. Hence, after several other parallelograms are formed then there is a
third triangle that is congruent to the other two. That is, triangle FEI is
congruent to GCD and ABH. See below:
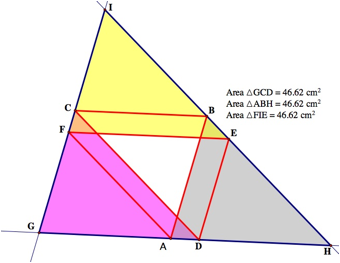
Because there are three congruent triangles and six
congruent parallelograms, Barney will always end at the same place. In the case
that Barney starts on any of the three segments then he will always end at the
starting point and he will hit a wall exactly five times.
Although, this is not always true if Barney starts at a midpoint, vertex, or triangle center.
If Barney starts his bouncing at a midpoint of one of the triangle segments he will always return to the same position after hitting two walls. During his travels, he hits the midpoints of each segments. I constructed the midpoints on the trianlgle below so one can see that he hits each midpoints. Thus, his path actually forms the medial triangle! Therefore, the distance of this pathway is exactly 1/2 of the distance traveled along the original triangle because the midpoints bisect the segments.
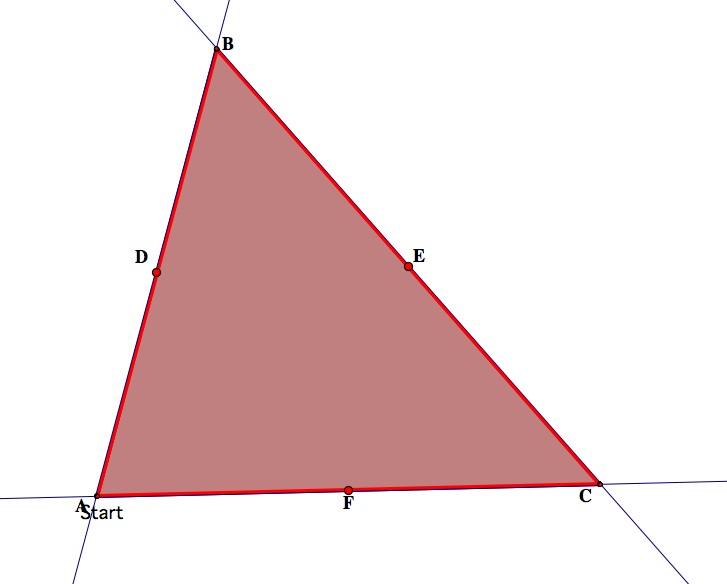
If Barney starts his travels at a vertex then would he return to his starting point?
Yes, simply because Barney travels along the perimeter of the triangle. This is the most starightforward case. He travels the distance of the perimeter of triangle ABC.
Interestingly enough, I have a conjecture about the distance traveled when Barney starts somewhere on the triangle, other than the midpoint because that has already been concluded. I believe Barney will travel a distance equal to the perimeter of the triangle. Using GSP we are able to conclude this. This is proved by the constructions of congruent parallelograms (highlighted in the first Barney exploration).
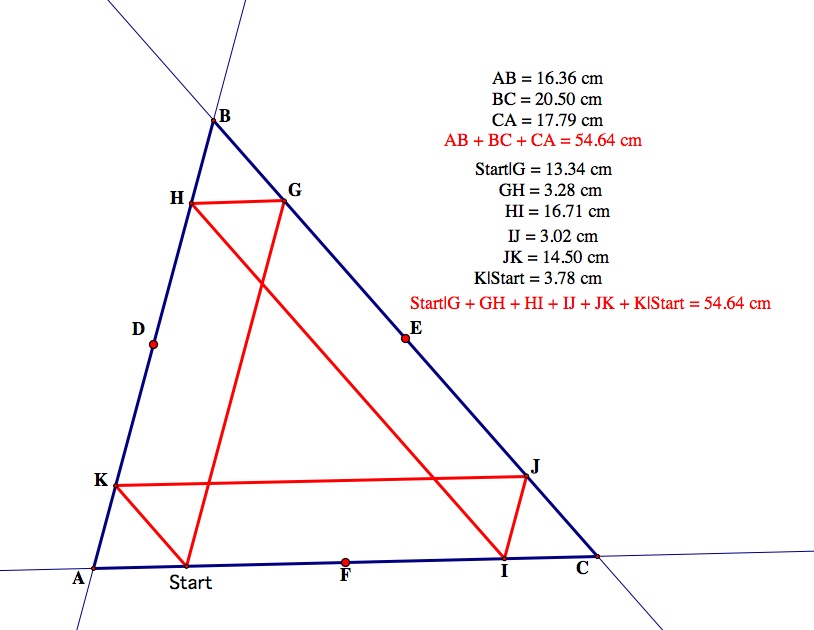
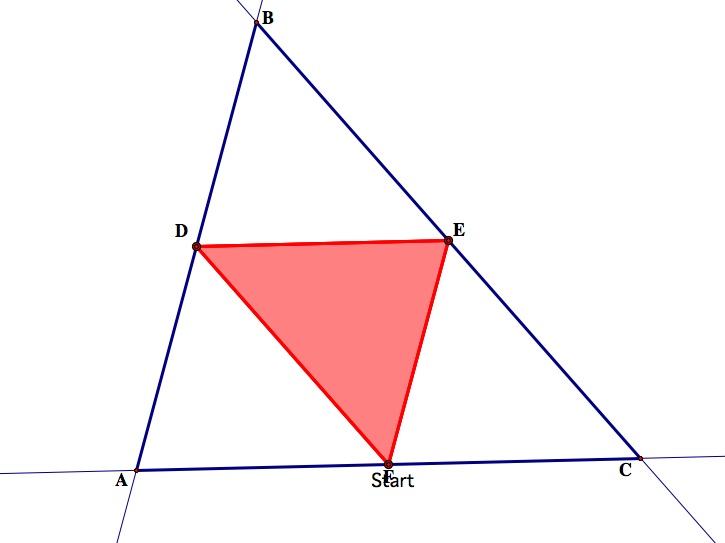
What if Barney starts at the centroid? This is the intersection of the three medians. Barney would start at the centroid and travel parallel to BC then hit at a point on AB. And that pattern continues until Barney returns to the centroid. Once he returns, he would have hit the wall twice and travled exactly 1/3 of the distance of the original triangle.